 with a vector function
with a vector function
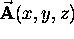 we get a vector result called the curl of
we get a vector result called the curl of 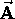 :
:
If we form the vector (``cross'') product
of  with a vector function
with a vector function
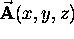 we get a vector result called the curl of
we get a vector result called the curl of 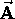 :
:

This is a lot harder to visualize than the divergence,
but not impossible. Suppose you are in a boat in a huge river
(or Pass) where the current flows mainly in the x direction
but where the speed of the current (flux of water) varies with y.
Then if we call the current  , we have
a nonzero value
for the derivative
, we have
a nonzero value
for the derivative 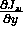 , which
you will recognize as one of the terms in the formula for
, which
you will recognize as one of the terms in the formula for 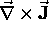 .
What does this imply? Well, if you are sitting in the boat,
moving with the current, it means the current on your port side
moves faster --- i.e. forward relative to the boat ---
and the current on your starboard side moves slower ---
i.e. backward relative to the boat --- and this implies a
circulation of the water around the boat ---
i.e. a whirlpool! So
.
What does this imply? Well, if you are sitting in the boat,
moving with the current, it means the current on your port side
moves faster --- i.e. forward relative to the boat ---
and the current on your starboard side moves slower ---
i.e. backward relative to the boat --- and this implies a
circulation of the water around the boat ---
i.e. a whirlpool! So  is a measure of
the local ``swirliness'' of the current
is a measure of
the local ``swirliness'' of the current  , which
means `` curl'' is not a bad name after all!
, which
means `` curl'' is not a bad name after all!