Conversion of Energy into Mass

In a nuclear reactor, a spontaneous nuclear process results in a net decrease in the net mass of all the particles involved. The ``missing mass'' appears as the kinetic energy of the reaction products, which is dissipated by what amounts to friction and generates heat that boils water; the steam is used to spin turbines that run generators that send electrical power down the wires.

This leads to an obvious question: can we do the opposite? Can we take electrical power out of the wires, use it to raise the kinetic energy of some particles to enormous values, smack the particles together and generate some extra mass? Yes! This is what a particle accelerator like TRIUMF does. Every such accelerator is a sort of ``reactor in reverse,'' taking electrical power out of the grid and turning it into mass.

Such things happen naturally, too. Gamma rays of sufficient energy often convert into electron-positron pairs when they have a glancing collision with a heavy nucleus. This is pictured below.

Figure: Electron-positron pair production by gamma rays (above) and by electrons (below). The positron (e⁺) is the antiparticle of the electron (e^-) [its opposite in every way]. The gamma ray (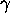) must have an energy of at least 1.022 MeV [twice the rest mass energy of an electron] and the pair production must take place near a heavy nucleus (Z) which absorbs the momentum of the .

There is a neat, compact way of representing such reactions by Feynman diagrams I will draw them ``left to right'' but the convention is actually to draw them ``down to up.'' I don't know why.

Figure: Feynman diagrams for pair production by a gamma ray (left) or an electron (right). These represent the processes in the preceding sketch.

The convention in Feynman diagrams is that antiparticle lines (e⁺, for instance) are drawn in the ``backward'' sense as if they were propagating backward in time. This allows all ``electron lines'' to be unbroken, a graphical expression of the conservation of electrons. There are lots more elegant graphical features to Feynman diagrams, but the details will have to wait for a course in quantum field theory.

The main point here is that the incoming particle(s) [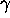 or e^-] must have at least 1.022 MeV of kinetic energy to create a positron and an electron, both of which have rest masses of 0.511 MeV/c². With an accelerator one can give the original projectile(s) more energy [there seems to be no limit on how much, except for mundane concerns about funding resources and real estate] and thus facilitiate the creation of heavier particles. At triumf, for instance, we accelerate protons to 520 MeV [just over half their rest mass energy of 938 MeV], which is enough to create mesons [mass = 139 MeV/c²] with reasonable efficiency; the high intensity of the TRIUMF cyclotron qualifies it for the elite club of `` meson factories,'' so named because they ``mass produce'' 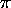mesons (or pions) in unprecedented numbers.

Figure: Feynman diagram for production of a 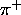meson by a collision between two protons (the most important interaction at TRIUMF).

Since heavier particles can in principle decay into lighter particles like gamma rays, neutrinos, antineutrinos, electrons and positrons, almost of these ``manufactured'' particles are unstable. Nevertheless, they hang around long enough to be studied and sometimes their very instability is what makes them interesting, if only because it precludes finding a cache of them in a more Natural setting.

Figure: Feynman diagram for `` associated production'' of a 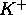meson [mass = 494 MeV/c<sup>2</sup> and ``strangeness'' S = +1] and a hyperon [a type of baryon with mass = 1193 MeV/c² and strangeness S = -1] in a collision between a 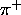and a proton (the pions produced at TRIUMF don't have enough energy to do this).

I have gotten far beyond my terms of reference here, but I wanted to ``preview'' some of the phenomenology of Elementary Particle Physics while focussing your attention on the simple motive for building higher- and higher-energy accelerators:

The more kinetic energy is available, the more mass can be created. The heavier the particle, the more options it is apt to have for other lighter particles to decay into, and the more unstable it can be expected to be; hence the less likely we are to observe it in Nature.

And the heavier the particle, the more exotic its properties might be.

So far this simple strategy has paid off in many new discoveries; of course, it may not keep working indefinitely....

Figure: Top: Feynman diagram for decay of a 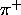meson [mass = 139 MeV/c²] into a positive muon (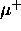) [mass = 106.7 MeV/c] and a [massless] muon neutrino (). Bottom: Feynman diagram for decay of a into a muon antineutrino (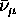), a positron () and an electron neutrino (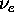). These are the reactions I use in almost all of my research.