BELIEVE ME NOT! -  - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE


Next: Captain Hooke
Up: Work and Energy
Previous: Work and Energy
Probably the most classic example of how the WORK AND ENERGY law
can be used is the case of a ball rolling down a frictionless hill,
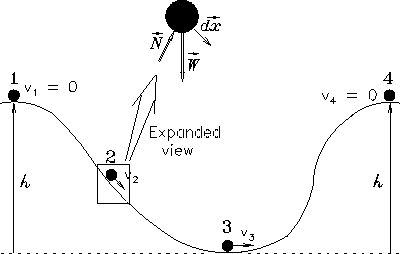
pictured schematically in Fig. 11.3.
Figure:
Sketch of a ball rolling down a frictionless hill.
In position 1, the ball is at rest.
It is then given an infinitesimal nudge
and starts to roll down the hill, passing position 2 on the way.
At the bottom of the hill [position 3] it has its maximum speed
v3 , which is then dissipated in rolling up the other side
of the hill to position 4. Assuming that it stops on a slight
slope at both ends, the ball will keep rolling back and forth
forever.
 |
Now, Galileo was fond of this example and could have given us a
calculation of the final speed of the ball for the case of
a straight-line path (i.e. the inclined plane); but he would
have thrown up his hands at the picture shown in Fig. 11.3!
Consider one spot on the downward slope, say position 2:
the FBD of the ball is drawn in the expanded view, showing
the two forces
 and
and
 acting on the mass m
of the ball.11.6
Now, the ball does not jump off the surface
or burrow into it, so the motion is strictly tangential to the hill
at every point.11.7
Meanwhile, a frictionless surface cannot, by definition,
exert any force parallel to the surface; this is why
the normal force
acting on the mass m
of the ball.11.6
Now, the ball does not jump off the surface
or burrow into it, so the motion is strictly tangential to the hill
at every point.11.7
Meanwhile, a frictionless surface cannot, by definition,
exert any force parallel to the surface; this is why
the normal force
 is called a "normal" force -
it is always normal [perpendicular] to the surface.
So
is called a "normal" force -
it is always normal [perpendicular] to the surface.
So
 which means that
which means that
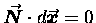 and the normal force does no work !
This is an important general rule.
Only the gravitational force
and the normal force does no work !
This is an important general rule.
Only the gravitational force
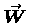 does any work on the mass m,
and since
does any work on the mass m,
and since
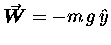 is a constant downward vector
[where we define the unit vector
is a constant downward vector
[where we define the unit vector 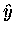 as "up"],
it is only the downward component of
as "up"],
it is only the downward component of
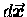 that produces any work at all. That is,
that produces any work at all. That is,
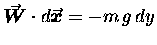 ,
where dy is the component of
d
,
where dy is the component of
d
 directed upward.11.8
That is, no matter what angle the hill makes
with the vertical at any position, at that position the work done
by gravity in raising the ball a differential height dy
is given by
directed upward.11.8
That is, no matter what angle the hill makes
with the vertical at any position, at that position the work done
by gravity in raising the ball a differential height dy
is given by
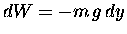 [notice that gravity does negative work going uphill
and positive work going downhill]
and the net work done in raising the ball a total distance
[notice that gravity does negative work going uphill
and positive work going downhill]
and the net work done in raising the ball a total distance 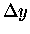 is given by a rather easy integral:
is given by a rather easy integral:
where 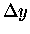 is the height that the ball is raised
in the process. By our LAW, this must be equal to
the change in the kinetic energy
is the height that the ball is raised
in the process. By our LAW, this must be equal to
the change in the kinetic energy
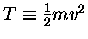 so that
so that
 |
(11.10) |
This formula governs both uphill rolls, in which 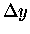 is positive and the ball slows down, and downhill rolls
in which
is positive and the ball slows down, and downhill rolls
in which 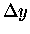 is negative and the ball speeds up.
For the example shown in Fig. 11.3
we start at the top with
v0 = v1 = 0 and roll down to
position 3, dropping the height by an amount h
in the process, so that the maximum speed (at position 3)
is given by
is negative and the ball speeds up.
For the example shown in Fig. 11.3
we start at the top with
v0 = v1 = 0 and roll down to
position 3, dropping the height by an amount h
in the process, so that the maximum speed (at position 3)
is given by
On the way up the other side the process exactly reverses itself
[though the details may be completely different!]
in that the altitude once again increases and the velocity
drops back to zero.
The most pleasant consequence of this paradigm
is that as long as the surface is truly frictionless,
we never have to know any of the details about
the descent to calculate the velocity at the bottom!
The ball can drop straight down, it can roll up and down
any number of little hills [as long as none of them are
higher than its original position] or it can even
roll through a tunnel or "black box" whose interior
is hidden and unknown -
and as long as I guarantee a frictionless surface
you can be confident that
it will come out the other end at the same speed as if it had
just fallen the same vertical distance straight down.
The direction of motion at the bottom will of course
always be tangential to the surface.
For me it seems impossible to imagine the ball rolling up and down the hill
without starting to think in terms of kinetic energy being stored up
somehow and then automatically re-emerging from that storage as fresh
kinetic energy. But I have already been indoctrinated into this way of
thinking, so it is hard to know if this is really a compelling metaphor
or just an extremely successful one. You be the judge.
I will force myself to hold off talking about potential energy
until I have covered the second prototypical example of the interplay
between work and energy.


Next: Captain Hooke
Up: Work and Energy
Previous: Work and Energy
Jess H. Brewer -
Last modified: Sat Nov 14 12:42:50 PST 2015
 - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE  - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE 