BELIEVE ME NOT! -  - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE


Next: Differential Equations
Up: "Solving" the Motion
Previous: Timing is Everything!
Let's write the equation of motion in a generalized form,
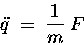 |
(12.7) |
where I have used "q" as the "canonical coordinate"
whose second derivative ( )
is the "canonical acceleration."
Normally q will be the spatial position x
[measured in units of length like metres or feet],
but you have already seen one case
(rotational kinematics) in which "q"
is the angle
)
is the "canonical acceleration."
Normally q will be the spatial position x
[measured in units of length like metres or feet],
but you have already seen one case
(rotational kinematics) in which "q"
is the angle 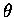 [measured in radians],
"m" is the moment of inertia IO
and "F" is the torque
[measured in radians],
"m" is the moment of inertia IO
and "F" is the torque 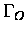 ;
then a completely analogous set of equations pertains.
This turns out to be a quite common situation.
Can we describe simply how to go about formulating
the equations of motion for "systems" that might even be
completely different from the standard objects of
Classical Mechanics?
;
then a completely analogous set of equations pertains.
This turns out to be a quite common situation.
Can we describe simply how to go about formulating
the equations of motion for "systems" that might even be
completely different from the standard objects of
Classical Mechanics?
In general there can be any number of canonical coordinates qi
in a given "system" whose behaviour we want to describe.
As long as we have an explicit formula for the
potential energy V in terms of one or more qi,
we can define the generalized force
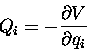 |
(12.8) |
If we then generalize the "inertial coefficient"  ,
we can write out
,
we can write out
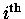 equation of motion in the form
equation of motion in the form
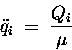 |
(12.9) |
which in most cases will produce a valid and workable solution.
There is an even more general and elegant formulation
of the canonical equations of motion which we will discuss
toward the end of this chapter.
I am not really sure how the term canonical
came to be fashionable for referring to this abstraction/generalization,
but Physicists are all so fond of it by now that
you are apt to hear them using it in all their conversations
to mean something like archetypal:
"It was the canonical Government coverup . . . " or
"This is a canonical cocktail party conversation . . . . "


Next: Differential Equations
Up: "Solving" the Motion
Previous: Timing is Everything!
Jess H. Brewer -
Last modified: Sat Nov 14 12:58:34 PST 2015
 - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE  - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE