BELIEVE   ME   NOT!    - -     A   SKEPTIC's   GUIDE  

The Electrostatic Force

First, what is a charge? We don't know! But then, we don't know what a mass is, either, except in terms of its behaviour: a mass resists acceleration by forces and attracts other masses with a gravitational force. The analogy is apt, in the sense that electrical charges exert forces on each other in almost exactly the same way as masses do, except for two minor differences, which I will come to shortly. Recall Newton's UNIVERSAL LAW OF GRAVITATION in its most democratic form: the force $\Vec{F}^G_{12}$ acting on body #2 (mass m₂) due to body #1 (mass m₁) is

$$\Vec{F}^G_{12} \; = \; - G \; {m_1 m_2 \over r_{12}^2} \; \hat{r}_{12}$$

where G is the Universal Gravitational Constant, r₁₂ is the distance between the two masses and $\hat{r}_{12}$ is the unit vector pointing from #1 to #2. The electrostatic force $\Vec{F}^E_{12}$ between two charges q₁ and q₂ is of exactly the same form:

$$\Vec{F}^E_{12} \; = \; k_E \; {q_1 q_2 \over r_{12}^2} \; \hat{r}_{12}$$ (17.1)

where k_E is some constant to make all the units come out right [allow me to sidestep this can of worms for now!]. Simple, eh?

  

Figure:  Comparison of the gravitational force $\Vec{F}_{12}^G$ on mass m₂ due to mass m₁ and the electrostatic (Coulomb) force $\Vec{F}_{12}^E$ on electric charge q₂ due to charge q₁.

This force law, also known as the COULOMB FORCE,^17.1 has almost the same qualitative earmarks as the force of gravity: the force is "central" - i.e. it acts along the line joining the centres of the charges - and drops off as the inverse square of the distance between them; it is also proportional to each of the charges involved. [We could think of mass as a sort of "gravitational charge" in this context.]

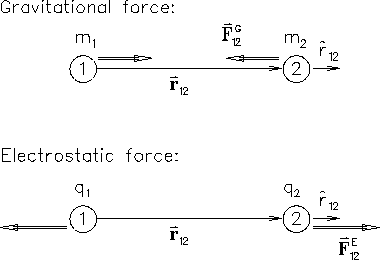So what are the "minor differences?" Well, the first one is in the sign. Both "coupling constants" (G and k_E) are defined to be positive; therefore the - sign in the first equation tells us that the gravitational force $\Vec{F}_{12}^G$ on mass #2 is in the opposite direction from the unit vector $\hat{r}_{12}$ pointing form #1 to #2 -- i.e. the force is attractive, back toward the other body. All masses attract all other masses gravitationally; there are (so far as we know) no repulsive forces in gravity. Another way of putting it would be to say that "there are no negative masses." By contrast, electric charges come in both positive (+) and negative (-) varieties; moreover, Eq. (1) tells us that the electrical force $\Vec{F}_{12}^E$ on charge #2 is in the same direction as $\hat{r}_{12}$ as long as the product q₁ q₂ is positive - i.e.

charges of like sign [both + or both -] repel
whereas unlike charges attract.

This means that a positive charge and a negative charge of equal magnitude will get pulled together until their net charge is zero, whereupon they "neutralize" each other and cease interacting with all other charges. To a good approximation, this is just what happens! Most macroscopic matter is electrically neutral, meaning that it has the positive and negative charges pretty much piled on top of each other.^17.2

The second "minor difference" between electrical and gravitational forces is in their magnitudes. Of course, each depends on the size of the "coupling constant" [G for gravity vs. k_E for electrostatics] as well as the sizes of the "sources" [m₁ and m₂ for gravity vs. q₁ and q₂ for electrostatics] so any discussion of magnitude has to be in reference to "typical" examples. Let's choose the heaviest stable elementary particle that has both charge and mass: the proton, which constitutes the nucleus of a hydrogen atom.^17.3 A proton has a positive charge of

$$e = 1.60217733(49) \times 10^{-19} \; \hbox{\rm coulomb (abbreviated C)}$$ (17.2)

[Don't worry about what a coulomb is just yet.] and a mass of

$$m_p = 1.6726231(10) \times 10^{-27} \; \hbox{\rm kg}$$ (17.3)

For any separation distance r, two protons attract each other (gravitationally) with a force whose magnitude F_G is ${G \; m_p^2 \over k_E \; e^2}$ times the magnitude F_E of the (electrostatic) force with which they repel each other. This ratio has an astonishing value of $0.80915 \times 10^{-36}$ -- the gravitational attraction between the two protons is roughly a trillion trillion trillion times weaker than the electrostatic repulsion. The electrical force wins, hands down. However, in spite of its phenomenal puniness, gravity can overcome all other forces if enough mass gets piled up in one place. This feature will be discussed at length later on, but for now it is time to discuss the basic magnetic force.