We can obtain the concomitant effect of Lorentz contraction
without too much trouble
using the following Gedankenexperiment, which is so simple
we don't even need a Figure:
Suppose a spaceship gets a nice running start and whips by the Earth
at a velocity u on the way to Planet X, a distance x away
as measured in the Earth's reference frame, which we call O.
[We assume that Planet X is at rest with respect to the Earth,
so that there are no complications due to their relative motion.]
If the spaceship just ``coasts'' the rest of the way at velocity u
[this is what is meant by an inertial reference frame],
then by definition the time required for the voyage is
t = x/u.
But this is the time as measured in the Earth's reference frame,
and we already know about TIME DILATION, which says that the
duration t' of the trip as measured aboard the ship
(frame O') is shorter than t by a factor of
:
.
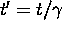Let's look at the whole trip from the point of view of the observer O' aboard the ship: since our choice of who is at rest and who is moving is perfectly arbitrary, we can choose to consider the ship at rest and the Earth (and Planet X) to be hurtling past/toward the ship at velocity u. As measured in the ship's reference frame, the distance from the Earth to Planet X is x' and we must have u = x'/t' by definition. But we also must have u = x/t in the other frame; and by symmetry they are both talking about the same u, so
and since
t =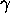 
t',
we must also have
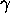x = x'.
That is, the distance between fixed points, as measured by
the space traveller, is shorter than that measured
by stay-at-homes on Earth by a factor of
.
This is because the Earth and Planet X represent the moving
system as measured from the ship. This effect is known as
Lorentz contraction; it has nothing whatsoever to do
with ``æther drag!''
Of course, the effect works both ways. The length of the spaceship, for instance, will be shorter as viewed from the Earth than it is aboard the spaceship itself, because in this case the length in question is in the frame that moved with respect to the Earth. The sense of the contraction effect can be remembered by this mnemonic:
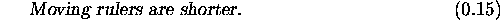
However, it is possible to conjure up situations that defy common sense and thus are often (wrongly) described as ``paradoxes.''