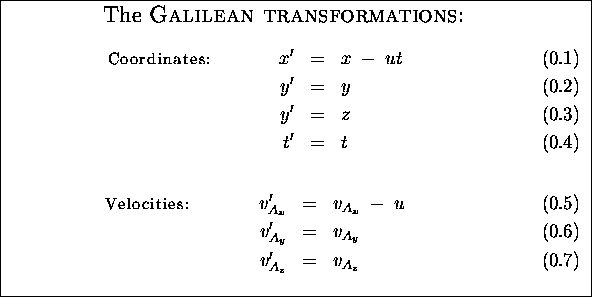Next: The Lorentz transformations
Up: No Title
Previous: No Title
So what? Well, this innocuous looking claim has some very
perplexing logical consequences with regard to relative velocities,
where we have expectations that follow, seemingly, from
self-evident common sense. For instance, suppose the propagation
velocity of ripples (water waves) in a calm lake is 0.5 m/s.
If I am walking along a dock at 1 m/s and I toss a pebble in the lake,
the guy sitting at anchor in a boat will see the ripples move by
at 0.5 m/s but I will see them dropping back relative to me!
That is, I can ``outrun" the waves. In mathematical terms,
if all the velocities are in the same direction (say, along x),
we just add relative velocities: if v is the velocity of
the wave relative to the water and u is my velocity relative to
the water, then v', the velocity of the
wave relative to me,
is given by v' = v - u.
This common sense equation is known as the
Galilean velocity transformation --
a big name for a little idea, it would seem.
With a simple diagram, we can summarize the common-sense
Galilean transformations
(named after Galileo, no Biblical reference):
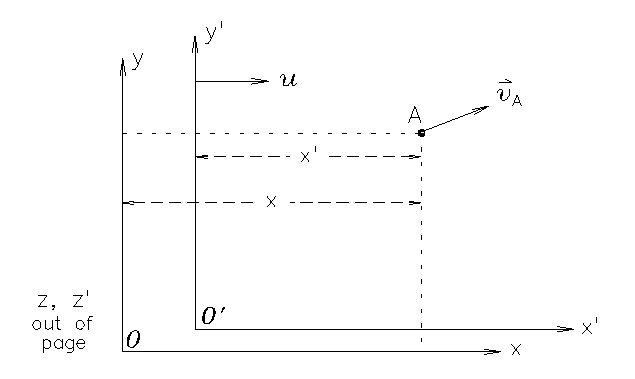
Figure 1:
Reference frames of a ``stationary" observer O
and an observer O' moving in the
x direction
at a velocity u relative to O. The coordinates
and time of an event at A measured by observer O
are {x,y,z,t} whereas the coordinates and time
of the same event measured by O' are
{x', y', z', t'}. An object at A moving at
velocity vA
relative to observer O will be moving
at a different velocity v'A in the
reference frame of O'. For convenience, we
always assume that O and O' coincide
initially, so that everyone agrees about the ``origin:" when
t=0 and t'=0, x=x', y=y' and z=z'.
First of all, it is self-evident that t'=t, otherwise
nothing would make any sense at all. Nevertheless, we include this explicitly.
Similarly, if the relative motion of O' with respect to O
is only in the x direction, then y = y'
and z = z', which were true at t = t' = 0,
must remain true at all later times.
In fact, the only coordinates that differ between the two
observers are x and x'. After a time t,
the distance (x') from O' to some obect A
is less than the distance (x) from O to A
by an amount ut, because that is how much closer
O' has moved to A in the interim.
Mathematically, x' = x - ut.
Nevertheless, we include this explicitly.
Similarly, if the relative motion of O' with respect to O
is only in the x direction, then y = y'
and z = z', which were true at t = t' = 0,
must remain true at all later times.
In fact, the only coordinates that differ between the two
observers are x and x'. After a time t,
the distance (x') from O' to some obect A
is less than the distance (x) from O to A
by an amount ut, because that is how much closer
O' has moved to A in the interim.
Mathematically, x' = x - ut.
The velocity v'A
of A in the reference frame of O
also looks different when viewed from O'
-- namely, we have to subtract the relative velocity
of O' with respect to O,
which we have labelled u.
In this case we picked u along x,
so that the vector subtraction
v'A = vA - u
becomes just
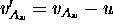 while
while  and
and 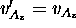 .
Let's summarize all these ``coordinate transformations:"
.
Let's summarize all these ``coordinate transformations:"
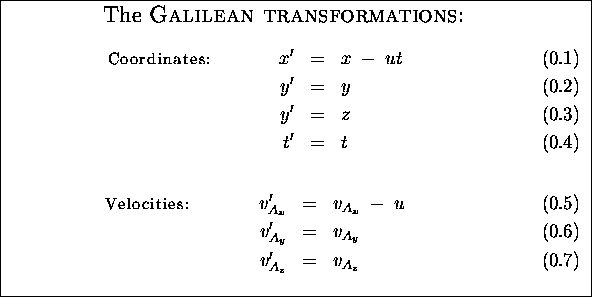
This is all so simple and obvious
that it is hard to focus one's attention on it.
We take all these properties for granted --
and therein lies the danger.



Next: The Lorentz transformations
Up: No Title
Previous: No Title
Jess Brewer
Fri Aug 16 17:01:55 PDT 1996

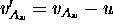 while
while  and
and 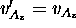 .
Let's summarize all these ``coordinate transformations:"
.
Let's summarize all these ``coordinate transformations:"