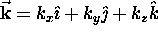with
,
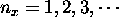etc.
Now there is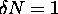 
allowed
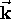for each
``k-volume'' element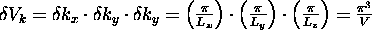 
,
where 
is the actual physical volume
of the three-dimensional box to which the waves are confined.
This gives a density of modes in k-space of
.
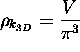In three dimensions, the extension is straightforward:
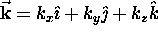with
,
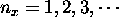etc.
Now there is 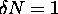allowed
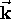for each
``k-volume'' element ,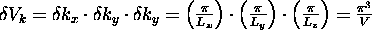
where is the actual physical volume
of the three-dimensional box to which the waves are confined.
This gives a density of modes in k-space of
.
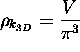The ``volume'' of k-space having wavenumbers within
dk of 
is now the positive octant
of a spherical shell of ``radius'' k
and thickness dk:
and this shell contains
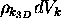allowed modes,
so the density of wavenumber
magnitudes (distribution function) in 3D k-space is
.
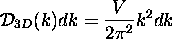Note that in this case the density increases as the square
of the wavenumber. In fact, we can generalize: if d is the
dimensionality of the region of confinement, then
.
In each case, the density of states in k-space is directly proportional
to the size of the real-space region to which the waves are confined.
More room, more possibilities.