BELIEVE ME NOT! -  - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE


Next: Changing Orbits
Up: Orbital Mechanics
Previous: Orbital Mechanics
The force and the acceleration are both centripetal
(i.e. back towards the centre of the Earth, so we can just
talk about the magnitudes of
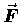 and
and
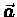 :
:
We can "solve" this equation for v in terms of r,
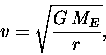 |
(10.6) |
or for r in terms of v:
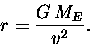 |
(10.7) |
You can try your hand with these equations.
See if you can show that the orbital velocity at the Earth's surface
(i.e. the speed required for a frictionless train moving through an
Equatorial tunnel to be in free fall all the way around the Earth)
is 7.905 km/s.
For a more practical example, try calculating the radius and velocity
of a geosynchronous satellite - i.e. a signal-relaying
satellite in an Equatorial orbit with a period of exactly one day,
so that it appears to stay at
exactly the same place in the sky all the time.10.10


Next: Changing Orbits
Up: Orbital Mechanics
Previous: Orbital Mechanics
Jess H. Brewer -
Last modified: Sat Nov 14 12:29:50 PST 2015
 - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE  - A SKEPTIC's GUIDE
- A SKEPTIC's GUIDE